4/15/2015: Magnetic Potential Energy Lab 13

Purpose: Demonstrate conservation of energy for a magnetic system.

Set up / Procedure:

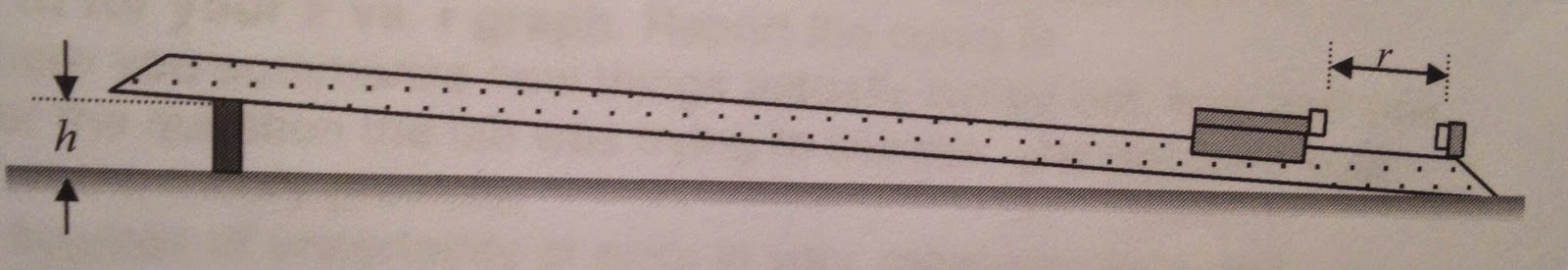
The set up consists of a level air track with a cart that has a strong magnet on one end and fixed magnet on the track of the same polarity. The track has tiny holes along its surface and an air source shoots air throughout the track, making the cart frictionless. 

For this experiment, we will be determining an equation for magnetic potential energy and verifying that conservation of energy holds true for this system. 

Part 1: Equation for magnetic potential energy

We begin by analyzing a cart when it is approaching the fixed magnet at some speed v. When the cart is closest to the magnet, the kinetic energy the cart had is momentarily converted and stored in the magnetic field as magnetic potential energy. The cart rebounds back and the magnetic potential energy is converted back into kinetic energy. The challenge is that we don't have an equation for magnetic potential energy, but we can find one by finding an equation for the force between magnets as a function of their separation, which we will can F(r) and then integrating F(r) to find U(r), which will be our equation for magnetic potential energy.

In order to find F(r), we will raise one end of the track using books so that the cart will end up at its position closest to the fixed magnet. At this equilibrium position, the magnetic repulsion force between the two magnets will equal the component force of gravity parallel to the track. We will vary ø, measure r (separation distance), and calculate F magnetic. We will then use logger pro to plot F vs. r in order to find a function of force in terms of r and integrate that function to get U(r).

We can calculate F magnetic by drawing a free body diagram when the cart is in its equilibrium position.

Below is a data table for F magnetic and r. We will plot these value in logger pro and use a power fit to find F(r).

As observed F(r) = Ar^n, where A = .0001092 n = -2.003

We can integrate this function to get a function for magnetic potential energy. Below is the derivation.

Part 2: Verifying conservation of energy for this system.

We will be using the same set up as in part 1, but adding a motion detector so we can determine the velocity of the glider. Level the track and with the air turned off, place the cart a reasonably close distance to the fixed magnet and run the motion detector. Determine the relationship between the distance the motion detector reads and the separation distance between the magnets. Below is an example how to calculate the separation. Next we set the motion detector to record 30 measurements per second. Finally, we can start with the cart at the far end of the track, turn the air back on, and hit collect on logger pro and give the cart a gentle push. Logger pro will generate data for position and velocity vs. time. 

Because the we want to verify conservation of energy, we can model our system by saying the KE(cart) + U(magnet) =  Total energy of the system.

We will create four calculated columns in logger pro for our system
mass of cart = .354 kg
distance from motion detector 0.341 m
distance between magnets 0.1 m

1. Seperation
2. KE of the cart
3. U magnetic
4. Total energy 

Below are calculated columns of how we entered the separation, KE, U magnetic, and total energy. 

We will need the position and velocity data logger pro generated when we gave the cart a gentle push in order to solve our four calculated columns. 

We can now verify conservation of energy for the time before, during, and after the collision. Below is our final data table showing how the energy remains almost constant throughout the carts motion. 

Below is a single graph showing KE, PE, and Total energy as a function of time. 

By analyzing our graph, we should see that the total energy should remain constant, therefore the total energy should be a horizontal line. What we generated was not horizontal, so there is some error in our experiment. The errors that could have deviated our total energy from being a horizontal line could have been: The uncertainty when measuring magnet separation, uncertainty when using our phones to measure the angle the track made with the table, there was an uncertainty in our fit for F vs r as shown in the image of our graph at the beginning, the air track may not be entirely frictionless, and the square plate on top of the glider could have contributed some air resistance during the carts motion towards the sensor. 

Conclusion: 

Our main purpose was to verify that conservation of energy holds true for a magnetic system. The challenge was that we didn't have a function for magnetic potential energy, but by using the connection between force and potential energy, we can say F = -du/dx, which means, the direction of force is opposite to the sign of du/dx. With this connection, we were able to determine the force between magnets as a function of their separation and then integrate that function to get the magnetic potential energy as a function of their separation. With a function for U magnetic, we can then verify conservation of energy for this system. Although our final graph was not perfect, we can still see the theory holds true.

4/13/15: Conservation of Energy Mass-Spring System Lab 12

Purpose: To verify that conservation of energy holds true for a vertically oscillating mass-spring system.

Procedure / Set up:

We will be performing two experiments: determining the spring constant and oscillating the spring to sum up the various energies involved in our system in order to find the total mechanical energy. 

Determining k

Mount a table clamp with a vertical rod to the table and to the vertical rod, mount a horizontal rod. Attach a force sensor to the horizontal rod and calibrate it using 1kg. Once calibrated, attach a spring to the force sensor and attach a hanging mass to the spring. Hit collect on Logger Pro and slowly pull down the mass so that the spring stretches. Logger pro will generate a graph of force vs. position and the slope of the graph will give us the value for k.

Our spring constant k was 8.057 N/m.

Stretch

We will need this value for the second part of this experiment because one of the various energies within our system is elastic potential energy in the spring, which includes the "stretch" as a variable. Set up a motion detector on the floor so it is right below the mass. Attach a notecard to the bottom of the mass so the motion detector can get an accurate reading. Adjust the spring so it is un-stretched and hit collect on logger pro to record the position of the mass. Our un-stretched value was 0.723 m

The stretch of the the spring = (un-stretched position - stretch position).

We will also need the mass of the spring which was .089 kg and the mass of the hanging weight which was 0.1 kg. These values will be used for the second part as well.

Total Mechanical Energy of the System

Part 2 will consist of the same set up as in part 1, only this time we will pull down the spring with the hanging mass attached and let it freely oscillate. We will verify that the total energy in the system is constant at any position. 

Various energies within the system, which will be input into logger pro as calculated columns:

EPE_spring­ = 0.5k(stretch)²

GPE_mass = (m_hanging)(“position”)g

KE_mass = 0.5(m_hanging)”velocity”²

GPE_{sping itself} = 0.5(M_spring)(“position”) We must include this as one of the energies in the system because the mass of the spring itself contributes to the potential energy of the system. Below is a derivation of how we can calculate this.

KE_{spring itself} = 0.5(M_spring/3)(V_end)² We must include the kinetic energy of the spring itself because the bottom end of the spring contributes to the kinetic energy of the system as it oscillates up and down. Using the same steps as in the image above, we can derive an equation for the KE of the spring itself. 

Now that we know our system will have 5 various energies, we can run the experiment and input our derived equations into calculated columns. We simply hung 250 grams of mass on the spring, pulled the spring down 10 cm hit collect and let it go. We made sure to record the position and velocity vs. time graphs because we will need those values for input into our calculated energy columns.

The main column we want to observe is the very last column, which is the total mechanical energy of the system.

TE = "EPE "+"GPE MASS"+"KE MASS"+"GPE Spring"+"KE Spring"

For the entire motion of the oscillating spring, the total energy should be constant.

We can verify this as well by summing the various energies at any point.

If we add up each energy at .58 seconds, the total energy is 0.985 J.

Below is a graph of the total energy.

In theory, the total energy graph should be a straight line, so it can be concluded that we have some error within our experiment. 

Possible sources of error:

The directions in the lab manual stated to hang 250 grams of mass when oscillating the spring, we didn't follow directions and hung 100 grams instead and only realized it until the end. 

As a result, the spring went past its equilibrium point when oscillating. Our equilibrium was 0.723 m, and the spring highest position above the ground was 0.732 m. Another source of error could have been made when measuring the equilibrium value of the spring. We measured the position with logger pro and then with a meter stick. Both values did not match. 

Conclusion:

The big picture of this lab was to verify that the conservation of energy theorem for a system holds true. To do this we stretched a spring over a distance and analyzed the graph logger pro generated in order to find the spring constant k. Our system included five various energies and by vertically oscillating a spring, we can see how these energies are transferred from one form to another throughout the motion of our system. We can also conclude that when the the mass reaches its lowest point, the elastic potential energy of the spring it at its max value. And when the mass reaches its highest point, the elastic potential energy of the spring is at its minimum value. We can also say that when the mass reaches its maximum and minimum height, the mass itself has no kinetic energy at that moment in time. Even though our results are not what was expected, we can conclude that all energy in the system was accounted for.